Class 12 Physics Periodic motion Notes

 

---

---

Unit - 1 

Mechanics

Chapter - 2

Periodic Motion

---

 

PDF Link:- View

Also Visit:-

• Class 12 Physics Notes
• Class 12 Notes
• Class 12 Practical File
• Class 12 Question Papers 
• Class 12 Important Questions

---

Simple Harmonics Motion

The motion of the body is said to be a simple harmonic motion if its acceleration is directed towards the mean position and is directly proportional to the displacement from that position.

i.e In Simple Harmonic Motion
acceleration ∝ displacement
acceleration = - k x displacement
where K is constant and the negative sign shows that acceleration is directed in opposition to the motion of an object.

Characteristics of Simple Harmonic Motion

(Relation between the acceleration and displacement of the particle executing S.H.M)

Fig: Body rotating in a circular path

Let us consider a body having mass 'm' is moving in a circular path of radius 'r' with constant speed 'v'. Suppose the body is initially at point A and after time 't', it reaches point B describing angular displacement 'Q'. Draw perpendicular BN on verticle diameter yy'. Also, Let which the particle at point B, the displacement by 'y' such that On = y

From figure
Sinθ = y / r
or, y = rsinθ --- (i)
If 'w' be the angular velocity then θ = wt
∴y = rsinwt --- (ii)

This is the displacement equation for a particle of S.H.M now, Velocity of S.H.M be,

This equation shows that velocity is not uniform

(i) When y = 0; v = wr

i.e at the mean position, the velocity is maximum.

(ii) When y = r; v = 0

i.e at the extreme position, the velocity is minimum.

Again, 

the acceleration of S.H.M be,

This equation shows the acceleration in S.H.M

(i) When y = 0; a = 0

i.e at the mean position, the acceleration is zero.

(ii) When y = r; a = -w²r

i.e at an extreme position, the acceleration is maximum.

Simple pendulum

A simple pendulum is a heavy point mass suspended by an inextensible weightless and flexible string from a rigid support and which is face to oscillate in a vertical plane.

Fig: Simple pendulum

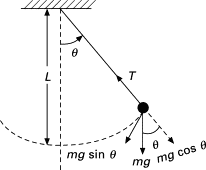
Let m be the mass of the bob of a simple pendulum and ‘l’ be its effective length (distance between the point of suspension to the C.G of the body) When the bob is not oscillating. The position of bob is called the mean position which lies at point ‘p’ below the point of suspension.

When the bob is displaced from its mean position it oscillates along the path OPQ. Also, let at any instant it be at point Q with angular displacement Q. The force on the bob at point Q is

(i)Weight may of the bob acting vertically downward

(ii) Tension in the string acting towards the point of suspension

The weight mg of the bob can be revolved into two components one is mgcosθ opposite to the tension and the other is mgsinθ towards the mean position. So mgcosθ balance tension provides restoring force.

So restoring force, F= -mgsinθ

For small θ

sinθ ≈ θ

∴ F= mgθ --- (i)

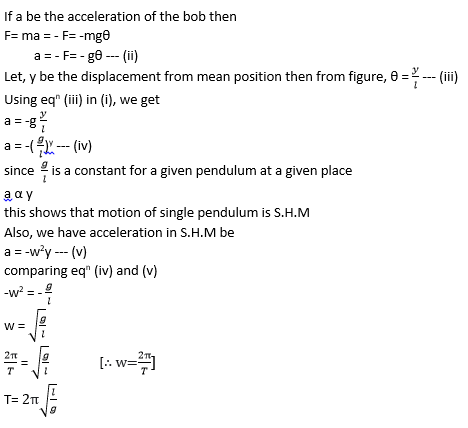
This is the required relation for a time period of a simple pendulum and this relation shows that the time period of a simple pendulum is independent of the mass of the bob but depended upon the effective length and acceleration due to gravity at that place.

Oscillation of a loaded spring

a. Vibration of a particle in horizontal spring

Let us consider a spring of negligible mass whose one end is attached to the wall other end is attached to an object of mass 'M'. The spring and the objects lie on the horizontal table as shown in the figure. When the mass is spring extends and lets 'L' be the elongation produced. Then by hooks law restoring force set up in the spring be,

F α l

F= -kl --- (i)

where k is a constant called force constant of spring force per unit extension, a negative sign shows that restoring force acts opposite to the displacement of mass.

If a be the acceleration produced on mass than,
F = ma --- (ii)
from equation (ii) and (i)
ma = -kl
a = -kl/m --- (iii)

This equation shows that acceleration is directly proportional to the displacement and is directed towards the mean position so the motion of horizontal mass. The Spring system is S.H.M
Also, we have acceleration is S.H.M be,
a = -w²l --- (iv)
Comparing equations (iii) and (iv) we get

This is a required expression for the time period of a particle in a horizontal spring.

(b) The vibration of a particle in verticle spring

Fig: Verticle Spiring

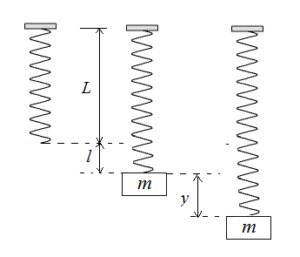
Let us consider a spring of force constant k suspended vertically from a rigid support. When a body of mass 'M' is attached to its lower end then suppose it is stretched by l. Then according to Hooke's law restoring force,

F1 = -kl = mg --- (i)

Let the cord is pulled down through a small distance y then the restoring force is given.

F2 = -k ( l + y ) --- (ii)

The effective restoring force which causes the oscillation is,

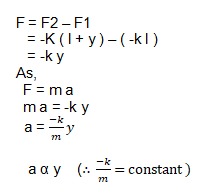
Hence, the motion of a loaded vertical spring is simple harmonic.

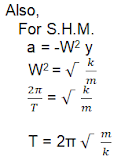
The time period of a mass-spring system depends on 

(1) Mass of the load attached
(2) Spring constant
(3) But the independence of acceleration due to gravity

Energy in S.H.M. 

For a body executing SHM, the restoring force acting on it cause its potential energy, and due to its motion, it exhibits kinetic energy. The energy of particle executing SHM in the sum of K.E. and P.E.

Consider a body executing SHM with amplitude 'r' and time 'T' with angular velocity W. Let 'm' be the mass of the particle. Now acceleration of the particle at any instant when its displacement from the mean position is 'y' is given by,

a = -W^2 y

So, the force acting on the particle

F = -m W^2 y

When a particle is displaced by small displacement 'dy' then small work done

dw = -F.dy [displacement and force are opposing]

= m W^2 y dy --- (i)

Now, the total amount of work done on the particle for the whole displacement is,

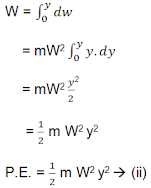
Again, the velocity of the picture at displacement y from a mean position is,

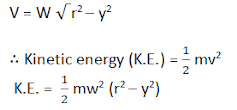
So, the total energy of the particle is given by,

This is the required relation for the total energy of a particle executing S.H.M. and this relation shows that total energy remains constant for a particle executing S.H.M.

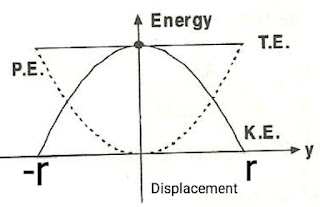
Case I

When the particle is at the mean position, y = 0

So, the total energy is equal to the maximum value of K.E.

Case II

When the particle is at the extremes position, y = r

So, the total energy is equal to the maximum value of P.E.
The variation of P.E and K.E with displacement in S.H.M is shown in the figure below,

Fig: Variation of K.E and P.E in S.H.M

Oscillatory motion

1. Damped oscillation

The oscillation in which amplitude gradually decreases with increases in time is called damped oscillation.

Fig: Damped oscillation

2. Sustained oscillation

The oscillation in which amplitude remains constant with time is called sustained oscillation.

Fig: Sustained oscillation 

Drawbacks of a simple pendulum

1. The string is considered to be weightless and inextensible but in practice, there is some weight of the string and some extension in the string when the bob is suspended.
2. The approximation sinθ≈θ is only θ＜1 but the experiment is usually performed with θ⋗ 1.
3. The bob is considered to the point mass but it is not so.

---

If you want to ask anything, please contact us by Messaging us on Social Media. 

 Social Media links:-

• Facebook
• Instagram
• Telegram
• Whatsapp

---

Tags

Class 12 Physics Notes All Chapters (New Curriculum)

Class 12 Physics Notes 2078: Complete Physics Guide (New Syllabus) | Questions Answers and Guide | Class 12 Physics Book Solution Guide  | Class 12 Physics Book PDF 2078 Solution | Class 12 Physics Book Notes 2078 Guide | Class 12 Physics All Chapter Notes | Physics Chapter Wise Notes. Class 12 Physics Rotational dynamics Notes.